Selecting an objective lens determines the field of view that can be observed at one time and the limit (spatial resolution) that can identify fine details. Also, in actual analysis, the size of the object to be measured can be divided into two cases: known and unknown. In each case, select the objective lens as follows.
1, When the size of the measurement object is known
Since the field of view and spatial resolution are decided for each objective lens, select the objective lens referring to it.
2, When the size of the measurement object is unknown
Advance measurement from low magnification to high magnification. After making a series of measurements, perform the main measurement by selecting the optimal magnification.
With the above in mind, let’s look at the relationship between magnification and numerical aperture (NA) of the objective lens and the field of view and spatial resolution.
※ The explanation about the numerical aperture (NA) is here.
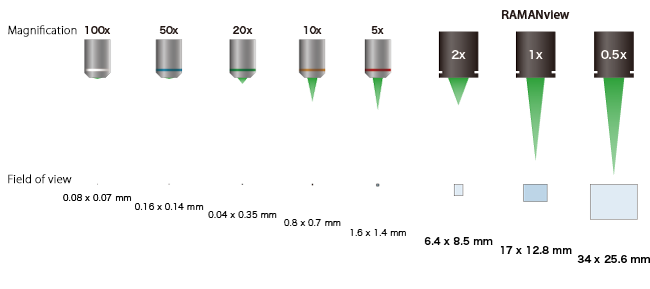
About the field of view for each objective lens
The field of view that can be measured is determined by the magnification of the objective lens. For example, the maximum field of view of the Raman image of the laser Raman microscope RAMANtouch is as shown in the table below. Therefore, select the magnification of the objective lens so that the object to be measured falls within the field of view.
▼Maximum field of view of Raman image for each objective
About spatial resolution for each objective lens
In confocal optics, the spatial resolution is determined by the numerical aperture N.A. of the objective lens, and the values differ between the planar (XY) direction and the depth (Z) direction. The theoretical formulas for spatial resolution in the planar and depth directions when using pinholes of the same spot size in confocal optics are shown below. The spatial resolution dxy in the planar direction is inversely proportional to N.A., and the spatial resolution dz in the depth direction is inversely proportional to the square of N.A.
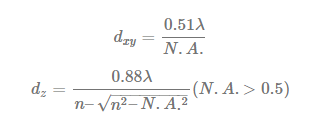
Here, n is the refractive index of the medium between the objective lens and the sample, and λ is the excitation wavelength. Because the numerical aperture NA is proportional to the refractive index n, both the planar and depth resolutions are inversely proportional to the refractive index n. Therefore, spatial resolution can be improved by using a water immersion lens or an oil immersion lens. The figure below shows the result of actually calculating the theoretical spatial resolution using the above equation (when λ = 532 nm, n = 1.0). When N.A. = 0.90, the spatial resolution in the theoretical plane direction is about 300 nm, and the resolution in the depth direction is about 830 nm.
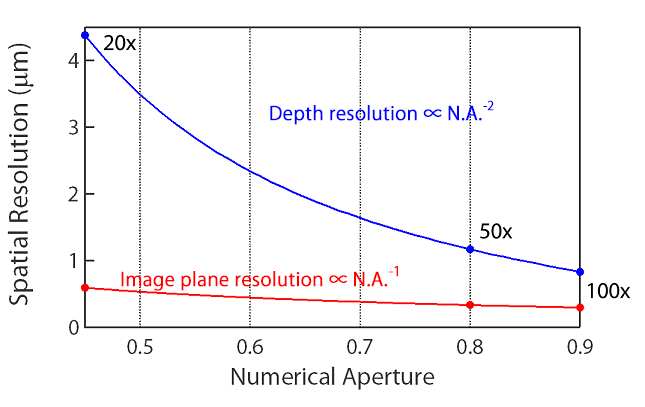
When measuring, if spatial resolution is not good enough for the size of the object to be measured, the object to be measured can not be determined, so spatial resolution is often prioritized over the field of view. Narrow vision is dealt with by dividing the measurement area into multiple times. Also, the tolerance for unevenness on the sample surface is based on the depth resolution. If the sample is a transparent sample or a sample with irregularities larger than the depth resolution, it is possible to obtain information in the depth direction by utilizing the features of the confocal optical system. That is, it is possible to perform cross-sectional (xz) imaging that repeats the line measurement by changing the focal position, or 3D imaging (xyz) measurement that repeats the planar imaging.
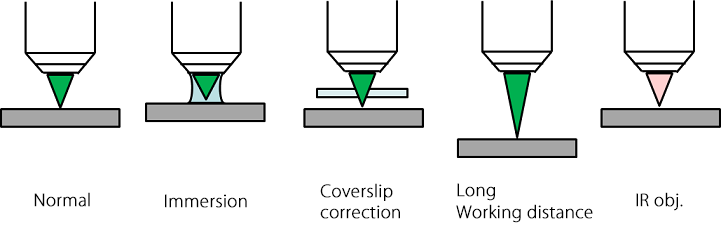
About special objective
Finally, if the sample is to be measured through glass or if it is in a special environment, such as in a solution, use a suitable objective. The following describes typical special objective lenses.
Immersion……Used when measuring samples in solution. In addition, the solution has a higher refractive index and improves the NA compared to when using a normal objective lens. Therefore, immersion lenses may be used to improve spatial resolution.
Coverslip correction……Used for measurement through the coverslip and observation glass window material. The thickness of the glass can be adjusted within the range of 0 mm to 1.2 mm.
Long Working distance……Because the working distance is long, it is used to measure uneven samples. It is also used for measurements in special sample environments, such as long working distance to protect the objective lens from heat during observation under heating.
For IR observation……When using a near infrared laser for the excitation wavelength, it is recommended to use a near infrared dedicated objective lens in view of the performance of the objective lens. Chromatic aberration and transmittance in the near infrared region are improved. Unlike the objective lens group above, it is not an objective lens applied to the environment around the sample.